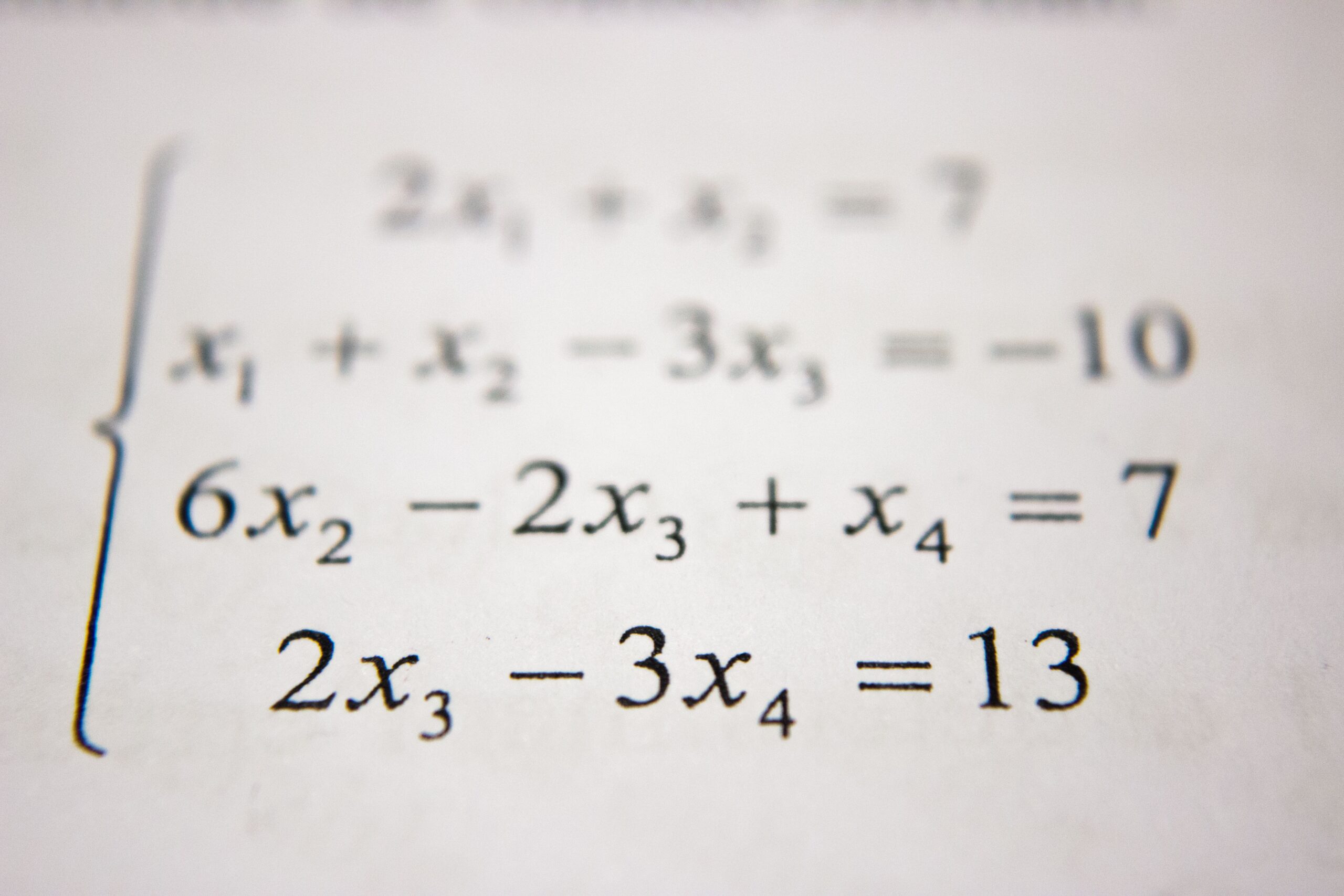Numbers are all around us, governing the very fabric of our existence. From the intricate calculations that chart the movements of celestial bodies to the simple arithmetic we use in everyday life, mathematics holds an undeniable power. In this blog post, we will delve into the fascinating world of numbers and explore some intriguing facts about mathematics. So buckle up and get ready to be amazed by the sheer power and beauty that lies within these numerical wonders!
The Meaning of “Hundred” Goes Beyond 100
Did you know that the meaning of “hundred” goes beyond its numerical value? Sure, we all learned early on in school that a hundred represents the number 100. But did you ever stop to think about the rich symbolism associated with this seemingly ordinary number?
In many cultures and languages, “hundred” is often used to represent abundance or completeness. It signifies a large quantity or an entirety of something. For example, when we say “a hundred percent,” we mean complete certainty or full commitment.
Interestingly, the concept of a hundred as a symbol for totality can be traced back to ancient civilizations. In numerology, where numbers are believed to possess spiritual significance, one interpretation suggests that a hundred represents completion and fulfillment.
So next time you come across the number 100, remember that it carries more than just its numerical value; it holds within it centuries of symbolic representation and conveys notions of abundance and wholeness. Mathematics truly has a way of weaving together artistry and logic!
The Only Even Prime Number
Did you know that the world of prime numbers is full of surprises? One fascinating fact about mathematics is that there exists only one even prime number. Can you guess what it is?
Well, let me tell you – it’s 2! That’s right, the humble number 2 breaks all expectations by being the only even number that can also be classified as a prime number.
Prime numbers are those special digits that can only be divided evenly by themselves and 1. Most people assume that all prime numbers must be odd, but 2 defies this convention.
Why is this so significant? Well, for starters, it means that every other even number can always be divided by 2 without leaving a remainder. This makes sense when we think about it because every even number has 2 as a factor.
But why does this matter in the grand scheme of things? It may seem like just another quirky mathematical anomaly, but understanding why 2 is the only even prime number helps mathematicians unravel deeper mysteries within number theory.
So next time someone asks you to name an even prime number (and really, who doesn’t love discussing math at parties?), impress them with your knowledge of the one and only – the enigmatic digit known as “two.”
The Intrigue of Pythagoras’ Constant: The Square Root of Two
Pythagoras, the famous mathematician and philosopher from ancient Greece, discovered a constant that still puzzles mathematicians today: the square root of two. This irrational number has an infinite decimal expansion that never repeats or terminates.
The discovery of the square root of two was groundbreaking because it challenged the prevailing belief in ancient Greece that all numbers could be expressed as fractions. The realization that there are numbers like this constant opened up a whole new world of possibilities in mathematics.
One fascinating aspect of the square root of two is its connection to right triangles. In a right triangle with sides measuring one unit each, the length of the hypotenuse (the side opposite the right angle) is exactly equal to the square root of two units. This relationship forms one cornerstone for understanding geometric concepts and calculating distances in various fields.
Despite its importance and intrigue, many people have never heard about this mysterious constant outside mathematical circles. But knowing about it can give you a deeper appreciation for how mathematics shapes our understanding and perception of reality.
So next time you encounter a right triangle or stumble upon discussions about irrational numbers, remember Pythagoras’ constant – an enigmatic symbol representing beauty and complexity within mathematics itself!
Zero’s Exclusion from Roman Numerals
Roman numerals, known for their unique system of representing numbers using letters, have fascinated people for centuries. However, one intriguing fact about Roman numerals is the absence of zero. Yes, you read that right – zero does not exist in this ancient numerical system!
In Roman times, numbers were represented by combining different letters to form a value. For example, I represents 1 and V represents 5. However, when it came to representing zero or nothingness, the Romans had no symbol for it.
The exclusion of zero in Roman numerals can be attributed to the culture and mindset of the time. The concept of nothingness was not widely embraced or understood during that era. Instead, they focused on tangible quantities and did not consider the need for a placeholder like zero.
Interestingly enough though, the concept of zero was already known in other civilizations such as the Mayans and Indians long before it found its way into mainstream mathematics.
The absence of zero in Roman numerals may seem puzzling today since we heavily rely on this digit in modern mathematical operations. However, it serves as a reminder of how our understanding and use of numbers has evolved over time.
So next time you come across a clock face with “IIII” instead of “IV”, remember that even though there might be no place for it within Roman numerals’ framework,
zero plays an essential role in shaping our current mathematical systems!
The Discovery of Zero Across Different Cultures
The concept of zero may seem simple and straightforward to us now, but its discovery was a profound breakthrough in mathematics. Interestingly, the concept of zero emerged independently in different cultures across the world.
In ancient Mesopotamia, around 300 BC, the Babylonians were using a placeholder symbol for an empty place value. However, it wasn’t until later that this placeholder evolved into the mathematical concept of zero.
Meanwhile, in India during the Gupta period (between the 4th and 6th centuries AD), mathematicians made significant advancements in understanding and using zero as a number. Indian mathematician Brahmagupta is often credited with formalizing rules for arithmetic operations involving zero.
On another continent entirely, ancient Mayans also had their own representation of zero. The Mayans developed a complex numeral system that included a shell-shaped glyph to represent zero.
It’s fascinating how these different cultures discovered and utilized the idea of nothingness independently. This speaks to the universal nature of mathematics and its ability to transcend borders and languages.
The discovery of zero laid down the foundations for more advanced mathematical concepts like negative numbers and decimals. It revolutionized calculations by introducing an essential placeholder that allowed for more precise counting and measuring.
Today we take for granted our ability to use zeros freely in everyday math tasks – from calculating bank balances to solving complex equations – thanks to those early pioneers who unlocked this powerful numerical tool.
Roman Numerals: Invented for Trade
Have you ever wondered why we use Roman numerals in certain contexts, like on clock faces or movie credits? Well, it turns out that these ancient numerical symbols were actually invented for a very practical reason – trade!
Back in the days of the Roman Empire, merchants needed a system to keep track of their goods and transactions. The Romans came up with an ingenious solution – using letters from their alphabet to represent numbers.
Each letter had a specific value assigned to it. For example, I stood for 1, V stood for 5, X stood for 10, and so on. By combining these letters and their values, they could easily represent any number they needed.
This system worked well because it was simple yet effective. Merchants could quickly calculate totals and make sure everything added up correctly. Plus, since the symbols were easy to write and recognize, there was less room for confusion or error.
Over time, this numbering system became ingrained in Roman society and spread throughout Europe as the empire expanded its influence. Even today, we still encounter Roman numerals in various aspects of our lives.
So next time you see those familiar I’s and X’s on a clock face or at the end of a movie credit roll, remember that they were originally created to facilitate trade thousands of years ago. It’s fascinating how something so practical has endured throughout history!
Zero: The Surprisingly Even Number
Let’s dive into the intriguing world of numbers and explore the fascinating fact about zero. While it may seem like an insignificant digit, zero plays a crucial role in mathematics and has some surprising characteristics.
What makes zero so unique is that it is an even number. In fact, it is the only non-negative integer that can be divided evenly by two! This might come as a surprise since most people associate evenness with numbers such as 2 or 4. But yes, zero fits the bill too!
Another interesting property of zero is its relationship with multiplication. Any number multiplied by zero equals…you guessed it, zero! It acts as a neutral element in multiplication, making calculations simpler and more efficient.
But perhaps one of the most mind-boggling aspects of zero is its place value system. Unlike other numerals that originated from various ancient cultures (such as Roman numerals), which didn’t include a symbol for representing nothingness or emptiness until later on, our modern numerical system includes the concept of zero from its very inception.
No matter how you look at it, whether through mathematical lenses or historical significance, there’s no denying that zero holds a special place in our numeric framework. So next time you encounter this seemingly insignificant digit while crunching numbers or pondering mathematical concepts, take a moment to appreciate just how surprisingly even this little numeral truly is!
The Lone Number with the Same Number of Letters as Itself
One number stands out from the rest, like a singular star in a vast mathematical universe. This captivating number is none other than “four.” Yes, you heard it right – “four” is the only number in the English language that has the same number of letters as its numerical value.
It may seem trivial at first glance, but this quirky fact highlights the fascinating nature of numbers and their connection to language. Four holds a special place among its peers, an anomaly that sparks curiosity and intrigue.
Imagine trying to find another number like four – you’ll quickly realize there’s nothing quite like it. Three comes close with five letters but falls short by two. Six also shares five letters but exceeds its value by one. No other digit can match four’s unique quality.
In this world where numbers reign supreme, four stands alone in its symmetrical beauty—a testament to mathematics’ inherent wonderment and endless possibilities.
So next time you encounter the number four, take a moment to appreciate its distinctive qualities—the lone number with the same number of letters as itself!
Read More:`Treating Water At Your Remote Bug Out Location, Survival Home
The Cool Trick of Multiplying Any Number by Three
Did you know that there’s a nifty little trick to quickly multiply any number by three? It’s like a secret mathematical hack that can save you time and mental energy. Let me show you how it works!
First, take the number you want to multiply by three. For example, let’s say we have 7. Now, all you have to do is double the number and then add the original number one more time.
So for our example of 7 multiplied by three, we start by doubling 7 which gives us 14. Then we add the original number again, so we get 14 + 7 which equals… drumroll please…21!
It may seem like magic at first, but it’s simply an elegant mathematical pattern. This trick works with any whole number – big or small.
Let’s try another example: multiplying 12 by three using our cool trick. Double twelve to get twenty-four and then add twelve once more. The result is thirty-six! Easy peasy, right?
This handy trick comes in handy when dealing with mental calculations or even on-the-spot estimations. Impress your friends with your lightning-fast multiplication skills!
Mathematics is full of fascinating tricks like this one that make numbers come alive in unexpected ways. So next time someone asks about multiplying a number by three, whip out this cool trick and watch their jaws drop!
Exploring the Magic of the Smallest Perfect Number: Six
Let’s dive into the enchanting world of numbers and uncover the magic behind one particular digit: six. While it may seem like an ordinary number, six holds a special place in mathematics as the smallest perfect number.
What exactly is a perfect number? Well, it’s a positive integer that is equal to the sum of its proper divisors. In simpler terms, when you add up all the numbers that divide evenly into six (excluding six itself), you get…six!
So, what are these proper divisors of six? They are 1, 2, and 3. And if we add them together – 1 + 2 + 3 – we indeed get our beloved number six! It’s like magic!
But here’s where things get even more fascinating. The concept of perfect numbers dates back thousands of years to ancient Greece. Mathematicians like Euclid and Pythagoras were captivated by these mystical digits and sought to understand their properties.
Interestingly, there are only a handful of known perfect numbers in existence today. As we explore further into this captivating realm, we’ll encounter larger examples such as 28,496 and 8,128 which also possess this intriguing property.
The study of perfect numbers continues to bewilder mathematicians worldwide who strive to unlock their secrets. So next time you come across the number six or any other seemingly ordinary digit on your math journey, remember that there might just be something extraordinary hiding beneath its surface! Keep exploring and embracing the power of numbers!
The Ancient Origins of Our Numerical System
As we have explored some fascinating facts about mathematics, it becomes clear that numbers hold immense power and significance in our lives. From the meaning of “hundred” to the intrigue of Pythagoras’ constant, from zero’s exclusion in Roman numerals to the discovery of zero across different cultures, each aspect reveals a unique facet of mathematics.
But where did our numerical system originate? It dates back thousands of years to ancient civilizations such as the Mesopotamians and Egyptians. These early mathematicians developed their own numeric symbols and counting methods, laying the foundation for what would become our modern system.
Over time, these numerical systems evolved and spread throughout various cultures. The Hindu-Arabic numeral system, with its use of place value and zero as a placeholder, emerged during medieval times. This revolutionary development transformed mathematics by making calculations more efficient and accurate.
Today, thanks to centuries of innovation and refinement, we enjoy a sophisticated mathematical framework that underpins countless aspects of our daily lives. From calculating finances to measuring distances or designing complex architectural structures – our numerical system is at work everywhere.
So next time you encounter numbers in your life or delve into mathematical concepts, remember the rich history behind them. Appreciate their power and marvel at how they shape our understanding of the world around us.
Numbers truly are remarkable entities that continue to captivate human curiosity as we strive to uncover even greater mysteries within the realm of mathematics – an ever-evolving field with infinite possibilities!